Definition of Trigonometric Functions
In the right triangle,
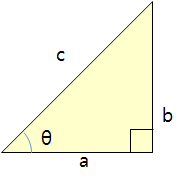
The longest side of the right triangle ‘c’ is the hypotenuse, ‘a’ is the base and ‘b’ is the height. You can assume that the angle between a and c is θ. Here is the definition of the classic trigonometric function:
As can be inferred from the word ‘trigonometric functions,’ the trigonometric function is a word derived from a triangle. So the trigonometric function seems to be most closely related to the triangle, and only the triangular shape seems to have meaning.
In practice, however, it is more important to understand trigonometric functions as the x- and y-axis components’ values over angles.
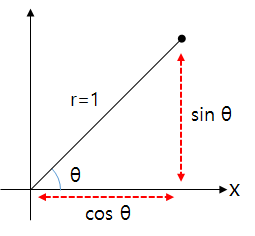
For example, suppose you have a line segment of length ‘1’ in a two-dimensional plane. If the angle between this line and the x-axis is θ, the coordinates at the end of the line (x, y) are (cos(θ), sin(θ)), respectively.
If the length of the line segment is variable ‘r,’ the coordinates of the end (x, y) of the line segment are (r·cos(θ), r·sin(θ)), respectively.
Application of Trigonometric Functions
Many trigonometric functions were used in the process of creating this ‘Java Lab.’
For example, when you need to rotate or circularly move an object on the screen, you have to use a trigonometric function to get the exact position.